
 |
|
With the usual caveats,1 and at risk of showing my great ignorance, I still don’t understand how gravity works. I’ve written about this before,2 and I read every popular explanation I can find, because the math-dense version is generally beyond me in all sorts of dimensions. And yet … some things about general relativity and gravity just don’t make sense to me.
Aristotle, the polymath and chief explainer of things scientific in the Greek golden age, thought gravity was simply the way that things find their own natural place. It’s linked to the concept expressed when we say that water seeks its lowest level. That is, gravity and its action on objects like draining pipes, falling stones, and stumbling people is merely a characteristic of the object itself. Water flows downhill, rocks tumble off cliffs, and you fall on your face if you trip, because that’s where the water, the rock, and you actually want to belong. That is, it’s the water’s and the rock’s fault—and yours.
Isaac Newton, who was the premier polymath of the 17th century, thought gravity was a force. Something about massive objects like the Sun and the Earth exerts a force to attract all lesser bodies, such as falling apples and those same stumbling humans. That is, gravity is a characteristic of the ground, not of the falling object itself. That is, it’s the Earth’s fault.
Newton’s concept of gravity worked well for a couple of hundred years and satisfied most of the observations of astronomy, governing the motions of stars and planets. The one problem was that Newton’s force of gravity was thought to be instantaneous: it was action at a distance not governed by time. So, if the Sun were to explode—no, that would still leave the equivalent mass in rapidly dispersing hydrogen, helium, and fusion products at the center of the solar system—or rather, the Sun could be magically “disappeared” from its central position, the Earth and the other planets would immediately head off in a straight line tangent to their normal orbits that had previously been shaped by gravity. In reality, at the speed of light—the limit governing all actions in the universe—the effects of any such instantaneous removal would take the same eight minutes to be felt at Earth’s orbit that it normally takes light from the Sun to reach us.
Albert Einstein, the polymath of the 20th century, rejected the idea of a “force” and, through his theory of general relativity, defined the effects of gravity as being a curvature in space and time. That is, massive objects bend space and slow down time. And the more massive the object, the more the surrounding space and time—which Einstein conceived as simply different dimensions of the same reality and called altogether “spacetime”—are curved. That is, it’s the fault of the geometry of space and time themselves.
In this conception, the idea of force and how quickly it might act or react is irrelevant, the curvature exists so long as the mass is present. And, of course, while the star might explode and scatter its mass, nothing known to physics is going to remove that mass, magically or otherwise, at any speed greater than, or in any timeframe shorter than, the speed of light, c.
As every science popularizer is quick to point out, Einstein’s concept of general relativity didn’t prove Newton “wrong.” Einstein’s concept of spacetime curvature and the mathematics to support it were just a more refined approach to the problem than a generalized force representing gravity. While Newton’s math worked for most problems in planetary astronomy—being useful, for instance, in calculating a near-Earth orbit or plotting a trip to the Moon—Einstein’s equations gave better answers to more decimal places. Einstein’s math, for example, gave a more accurate prediction of the precession of Mercury’s orbit around the Sun than Newton’s by a few seconds of arc.
Still, and mathematics aside, Einstein and Newton offer very different and irreconcilable conceptions: Newton postulates a force whereby one body acts upon another, like a pitcher hurling a baseball;3 while Einstein postulates the effect that a massive body has on its surroundings, and that effect is present regardless of whether any second body is around to experience it.
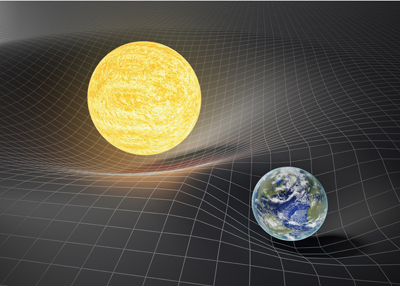
For ease of visualization by the layperson, illustrators show the curvature effects of gravity under general relativity with something like a bowling ball sitting on a trampoline and creating a curved depression in the surface—like the illustration here. The trampoline is supposed to represent the “fabric of space.” Of course, the curve is not in just the two dimensions shown for this flat surface but in all three dimensions of space plus a commensurate slowing of time.
I have always had a problem with this usage, even as an analogy, of the word “fabric” to refer to space and time. Space in all other contexts, is generally accepted as simply being empty. If it has a structure, an internal component that can be bent or warped, then space is not just a form of emptiness but instead is something all its own and separate from the protons, neutrons, electrons, photons, and other particles that exist within it and pass through it. Similarly, if time can be made to slow down, that implies some structure or medium that a nearby mass somehow manipulates. Time is not just the measured passage of events but a thing all its own, separate from those events.
In quantum mechanics, particles have associated fields, and these fields guide the motions of the particles within them. The photon, for example, is not just tiny, massless “thing,” but it also establishes a field that guides the movement of light and the properties of magnetism. Supposedly, in quantum mechanics, there must exist a particle called a “graviton” that has an associated field governing what we experience as gravity. But such a particle has never been discovered.4 Otherwise, we could hope to make our cars fly by blocking the exchange of gravitons with the Earth beneath them. But no one has yet been able to reconcile the concepts and mathematics of quantum mechanics with general relativity. Big is big, small is small, and they don’t seem to play by the same rule sets as currently conceived by the best human minds.
The confusion I have with general relativity and the curvature of spacetime is this: I can understand how curved space and time might alter the direction of a body that is already in motion, such as planet Earth wanting to move in a straight line (like all good inertial objects) but being forced into an elliptical orbit by the mass of the Sun. But what about a body that is not moving with respect to that center of mass? Just sitting or standing on the surface of the Earth, I am—according to general relativity—accelerating toward the center of the planet. But I am not moving with respect to that center. I never get any closer to the center, although I am accelerating toward it at a rate of 9.8 meters—more than 32 feet—per second per second. That would be a pretty good clip if I were moving across the surface of the planet and going faster and faster with every second.
Sure, the analogy with a trampoline shows a depression that I might be sliding into, like a kid on a sled sliding “down” a hill. But if I am at rest with respect to the center of the planet or another nearby mass, why would I be moving toward it at all? Even if that surrounding space is curved, what … forces me to move down the curve?5
I’ve read explanations that all of this has to do with different and higher orders of geometry. Also, that objects existing in a faster timeframe, such as in the less-curved spacetime further away from a planet or star, will seek to move toward the slower timeframe created by the mass of a large nearby body. Perhaps it all works out with elegant mathematics. But that still leaves the common-sensical question of why an object would prefer, and naturally move toward, a slower timeframe. Isn’t that just a version of Aristotle’s definition of gravity: that things just try to find their natural place?
I don’t mind if there’s math involved. That doesn’t insult or frighten me. But I do mind if the concept is solely based on mathematical equations. If the underpinnings of the universe cannot be explained except through a set of equations, then we run the risk of the ever-inventive and fertile human mind creating an equation that describes a situation without actually explaining it.6
You can write any number of equations, and they may make mathematically perfect sense. I can measure the distance across the continental Unites States in terms of gallons of milkshake consumed at Dairy Queens along the way. I can relate this function to a traveling body’s metabolism and the ambient temperature, and then link that intake to toilet flushes in restrooms further down the road. I can create elaborate mathematical structures related to distance and dairy products. But they won’t explain anything.
I still don’t understand gravity. And given that we have to fudge around with concepts like “dark matter” to reconcile current conceptions of gravity with the observed motion of stars in galaxies, and with “dark energy” to relate the motions of those galaxies with the size and scale of the universe itself … I don’t think anyone else does, either.
1. I was an English major in college with a minor in karate. The highest level of math I took in high school was Algebra II and Geometry, and I satisfied my college math requirement, as did so many other liberal arts students, with Philosophy I (aka Logic). But since then I’ve been reading continuously in the sciences, particularly physics and astronomy, to support my science fiction writing. My professional life over the years has been to explain the work of engineers and scientists for the lay reader. So, while I am math-challenged, I am neither ignorant of nor disinterested in the subject.
2. See Three Things We Don’t Know about Physics (I) from December 30, 2012, and (II) from January 6, 2013.
3. A force is represented by the most basic equation in physics, f=ma, or “force equals mass times acceleration.” The pitcher’s arm muscles accelerate the 142-gram mass of a baseball from, say, zero miles per hour in his set position to, say, 90 miles per hour—or 132 feet per second—for a fastball at the full extension of his arm at release, which occurs about half a second later. That’s an acceleration—not an exit velocity, but the acceleration needed to achieve it—of 264 feet per second per second. Mass times acceleration. Einstein used a variation of this physics equation to come up with his famous statement about the energy content of matter itself, e=mc2.
4. Recently, there was much to-do when the Large Hadron Collider at CERN identified the previously theoretical Higgs boson. This heavy particle, which is not normally found in nature, is supposed to give matter its mass. This is a different particle from, but might be a kind of precursor to, a graviton. We still have much to learn.
5. I used this question to create a fantasy mechanism for time travel in The Children of Possibility.
6. I can define gravity as the hand of an ever-watchful and invisible little god, call him “Mr. G.” He watches me and every other thing in the universe. If I am sitting, he presses gently on my lap so that I don’t float away from the seat of the chair. If I am walking and careful about my steps, he has a hand on my shoulder to keep me in contact with the ground. But if I stumble, he flicks the back of my head with his finger, pushes me over, and presses me down. If I jump, he lets me rise only so far, consistent with my muscle tone, and then pushes me back down to the floor. And if I take a capsule into orbit, he watches my direction and speed, and at the appropriate time he stops pushing down on me so that I can float freely around the cabin. There! I have a working concept of gravity that fits all observations. I could even write out Mr. G’s influence in the form of a set of equations. But is this what’s actually going on in the universe?